Absolute value

Terminology and Notation
– Jean-Robert Argand introduced the term ‘module’ in 1806, meaning ‘unit of measure’ in French.
– The term ‘module’ was borrowed into English in 1866 as the Latin equivalent ‘modulus’.
– The term ‘absolute value’ has been used since at least 1806 in French and 1857 in English.
– Karl Weierstrass introduced the notation x, with a vertical bar on each side, in 1841.
– Other names for ‘absolute value’ include ‘numerical value’ and ‘magnitude’.

Definition and Properties
– For any real number x, the absolute value or modulus is denoted by |x|.
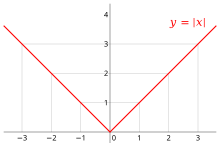
– The absolute value of x is either x or -x, depending on whether x is greater than or equal to 0 or less than 0.
– The absolute value is always a positive number or zero, never negative.
– The absolute value represents the distance of a real number from zero on the number line.
– The absolute value function is continuous everywhere and differentiable everywhere except at x = 0.

Real Numbers
– The absolute value of a real number x is denoted by |x|.
– The absolute value of x is x if x is greater than or equal to 0, and -x if x is less than 0.
– The absolute value is always a positive number or zero, never negative.
– The absolute value represents the distance of a real number from zero on the number line.
– The absolute value of the difference between two real numbers is the distance between them.

Complex Numbers
– The absolute value of a complex number z is denoted by |z|.
– The absolute value of z is the distance from the origin.
– The absolute value of a complex number and its complex conjugate have the same absolute value.
– The absolute value of a complex number is the square root of the product of the number and its conjugate.
– The absolute value of a complex number is continuous everywhere but complex differentiable nowhere.

Absolute Value Function
– The graph of the absolute value function for real numbers is continuous everywhere.
– The absolute value function is monotonically decreasing on the interval (-∞, 0) and monotonically increasing on the interval (0, +∞).
– The absolute value function is an even function and is not invertible.
– The absolute value function is idempotent, meaning that the absolute value of any absolute value is itself.
– The absolute value function is a piecewise linear, convex function. Source:  https://en.wikipedia.org/wiki/Absolute_value

Absolute value (Wikipedia)

This article is about the absolute value of real and complex numbers. For other absolute values in mathematics, see Absolute value (algebra). For other uses, see Absolute value (disambiguation).

In mathematics, the absolute value or modulus of a real number ${\displaystyle x}$, denoted ${\displaystyle |x|}$, is the non-negative value of ${\displaystyle x}$ without regard to its sign. Namely, ${\displaystyle |x|=x}$ if ${\displaystyle x}$ is a positive number, and ${\displaystyle |x|=-x}$ if ${\displaystyle x}$ is negative (in which case negating ${\displaystyle x}$ makes ${\displaystyle -x}$ positive), and ${\displaystyle |0|=0}$. For example, the absolute value of 3 is 3, and the absolute value of −3 is also 3. The absolute value of a number may be thought of as its distance from zero.

Generalisations of the absolute value for real numbers occur in a wide variety of mathematical settings. For example, an absolute value is also defined for the complex numbers, the quaternions, ordered rings, fields and vector spaces. The absolute value is closely related to the notions of magnitude, distance, and norm in various mathematical and physical contexts.